
IV. Ore systems and processes
Distribution of rare an rare-earth elements in the fluid magmatic systems at high pressures
Volatiles are the main factor of the processes of rock and ore formation and rare (RE) and rare-earth (REE) elements are their sensitive genetic indicator. Their behavior in the magmatic processes is governed by l-m-f (melt- mineral- fluid) distribution. Short results of the experimental study on the latter are presented below.
# Zharikov V.A., Gorbachev N.S. Distribution of rare-earth elements between fluid and melt.
a) The effect of P, T, composition, melt.
Experimental study on the distribution of rare-earth elements and yttrium between basalt and lamproite melts and the solutions (H2O, H2O+HCl) has been carried out in the temperature range 1100-1300oC and pressures of 1-14 kbar. It was found that in the basalt system distribution coefficients Df/l increase from La to Lu by a half an order and in the lamproite system in the light REE increase by an order and more, in the series of heavy REE they change insufficiently. A good correlation between Df/l of REE and their affinity to oxygen, chlorine, REE volatility is observed. Pressure dependence of REE Df/l (Fig.1) in the pressure range 1-9 kbar is of extreme character, at 5 kbar a distinct minimum is observed. In the pressure range 9-14 kbar Df/l of REE change in narrow limit. Df/l of REE increase insufficiently with decreasing temperature from 1200 to 1100oC. The results of the study on the l-f distribution of REE testify to the possibility of the REE fractionation at the magmas degazation which is comparable with their fractionation at the crystallization differentiation (Zharikov et al., 1993).

Fig.1. Dependence of the distribution coefficients of rare-earth elements and yttrium on pressure at T=1200oC.
b) Acid-basic interaction and fractionation of Eu.
Eu participates in the endogenic processes in the form of Eu+2 and Eu+3 differing in the acid-basic properties. Distribution of Eu in the l-m-f systems is governed both by redox conditions and acidity-basicity of the fluid. So, at T=1100oC, P=5 kbar, NNO buffer a distinct relative maximum of Eu distribution in favor of acidified solutions was determined (Fig.2). Existence of the maximum is largely connected with the Eu+2 dissolution in the acidified solutions. In the light of these data minimum in the granitoids may be related to the effect of acidified fluids of postmagmatic and metamagmatic stages (Zharikov, Gorbachev, 1993).
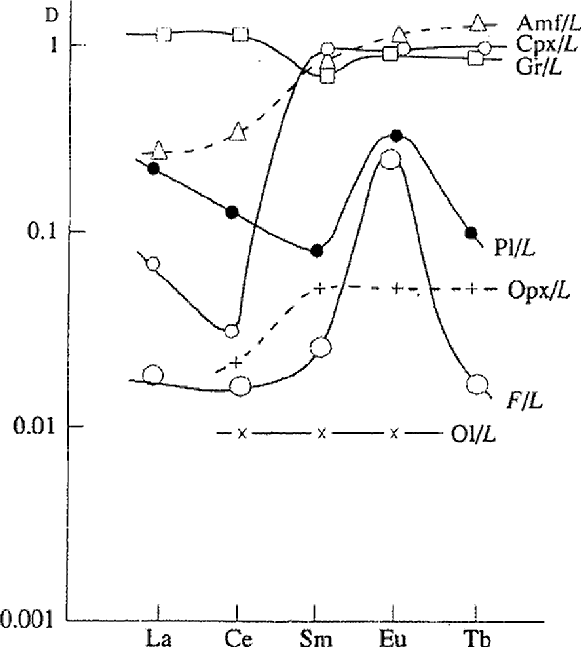
Fig.2. Distribution coefficients of rare-earth elements between fluid and basalt melt (our data) and rock forming minerals (literature data). Symbols: F - fluid, L - melt, Ol - olivine, Opx - orthopyroxene, Cpx - clinopyroxene, Pl - plagioclase, Gr - garnet, Amf - amphibole.
c) Modelling of crust contamination of basalt magmas: distribution of REE and Y.
The study on the distribution of REE and Y in the basalt melt at its interaction with anhydrite-dolomite-marl rocks at T=1100oC, P=5 kbar at "wet" (fluid excess) and "dry" ( sandwich method, with natural water content) conditions showed that basalt melt was enriching in REE and Y as compared to the starting composition, preferentially in MREE (medium REE) and HREE ( heavy REE) and to the less degree in LREE (light REE) which suggests the anisochemical character of the processes of crust contamination of basalt magmas (Gorbachev, Lightfoot, et al., 1994).
d) Distribution of REE and Y between sulfide and basalt melts.
REE and Y are characterized by, as a whole, low and different affinity to the sulfide melt. At T=1200oC, P=5 kbar, CCO and QFM buffers, D of REE and Y vary from n× 10-3 to n× 10-1, decreasing from LREE to HREE and Y
30
from odd to even REE and increasing with fO2 growth (Fig.3). The possibility of REE fractionation at the sulfide-silicate layering is substantiated. On the example of the effusives of the Norilsk region the efficiency of the relations RE and REE (Sm/Eu, Nd/Sm, Gd/Tb, Pt/Y, Pd/Y, Cu/Y) as geochemical indicators of the sulfide control at the generation and evolution of basite magmas and formation of the sulfide-bearing magmatic complexes was shown (Gorbachev et al., 1995).

Fig.3. Distribution coefficients of rare-earth elements between sulfide and basaltic melt at T=1200oC, P=5 kbar. Symbols: 1 - QFM, 2 - CCO buffer.
# Gorbachev N.S., Khodorevskaya L.I. Distribution of Cl, ore elements (Cu, Zn, Pb, Fe) and noble metals between fluid and magmatic melt of basic and medium composition.
a) Distribution of Cl. In the range T= 1100-1300oC, P=1-12 kbar D of Cl between fluid (H2O+HCl, H2O+HCl+CO2) and basalt and andesite melts varies from 2 to 7, and solubility from 0.6 to 1.8 wt%. D of Cl increases with temperature drop, with an increase of Cl concentration in the fluid and reaches the maximum at P=5-6 kbar (Fig.1).
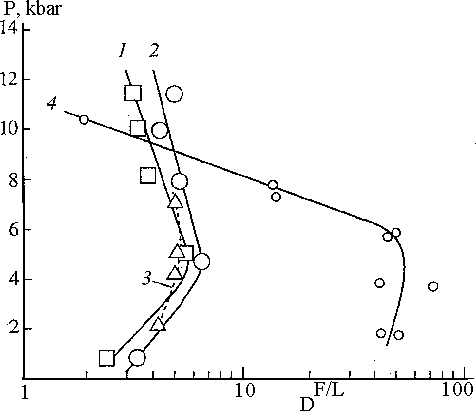
Fig.1 Pressure dependence of the fluid melt partition coefficients of Cl.
Symbols: 1,2-tholeiitic basalt +1N HCl (1), +1N HCl+1.5 m CO2 (2), 3-andesite+1N HCl, 4 - granite+0.75 mNaCl+0.45 m Kcl.
Based on experimental and geochemical data a model has been developed on the degazation of the Earth upper mantle. It was shown that H2O and Cl fractionation did not take place in the process of degazation. The ratio H2O/Cl in the upper mantle, primitive basalt melts, juvenile fluid, and oceanic water are similar. Therefore, Df/lCl=Df/lH2O (Gorbachev, Khodorevskaya, 1995).
b)The effect of pressure on the distribution of Fe, Cu, Zn, Pb between fluid and basite melts: fluid-melt geobarometry of magmatic degazation. Coefficients of distribution (D) and partition (KD) of elements between fluid and melt (H2O+Cl, H2O+HCl+CO2) and basalt and andesite melts at T=1100oC, pressure range 1-12 kbar varies from n× 10-2 to n. Pressure dependence Df/1 is of extremal character with the maximum at 4-5 bar, KD f/l increase with pressure (Fig.2). Based on the experimental data on K-P dependencies a series of geobarometers has been developed for the estimation of the depth of formation of ore bearing fluids from the pyrite deposits of massive sulfide ores (Khodorevskaya, Gorbachev, 1993; Gorbachev, Kashirtseva, Naldrett, 1994).
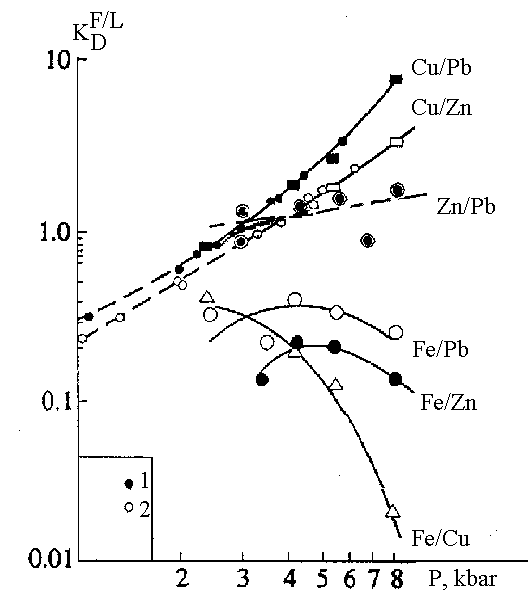
Fig.2. Pressure dependence of the exchange coefficient of metals between fluid and andesite melt.
c) Distribution of PGE (Platinum Group Elements) and Au between water-chloride fluid and basalt melt. Experimental data on the solubility and distribution of PGE and Au in l-f systems showed a high ore generating ability of the fluid-bearing basalt magmas and efficiency of the fluid transfer in mobilization and transfer of noble metals. In the temperature range 850-1300oC Au solubility in the fluid varies from 15 to 200 and more ppm, in the melt to 20 ppm, Pt in the fluid varies from 150 to 700 ppm, in the melt to 250 ppm. Df/l of PGE and Au varies from n× 10-2 to
#This investigation was supported by RFBR N 97-05-65925, 96-05-64871.
31
n (Fig.3). The correlation between Df/l of the noble metals and their affinity to Cl allows to predict Df/l of chlorotype metals (Gorbachev, Naldrett et al., 1994).
d) The effect of oxidation conditions on the distribution of PGE between sulfide and silicate melts. The study of the effect of redox conditions (WM, NNO, MH buffers) on the distribution of Rh and Pd between sulfide and basalt melts at T=1250oC and P=5 kbar showed an important role of fO2 in this distribution. With an increase in fO2 Dsl/l grows by 1 - 1.5 order (Fig.4). The character of Df/l PGE on fO2 dependence is related to siderophile and chalcophile properties of metals. In this connection the distribution of PGE in the primitive mantle, lavas and sulfide ores of the Norilsk region has been considered (Gorbachev et al., 1993).

Fig.3. Partitioning of the PGE and Au between fluid (1 m HCl) at T=1100oC , P=5 kbar.
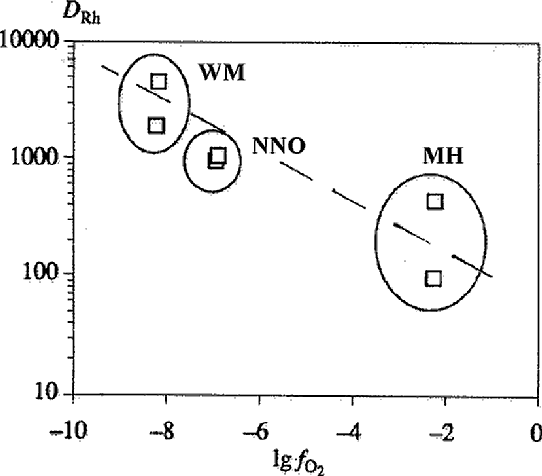
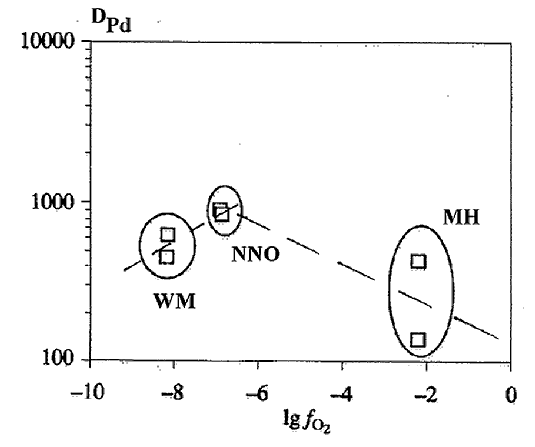
Fig.4. The effect of fO2 on the distribution of PGE between sulfide and silicate melts.
References:
32
mental data). // Dokl. RAS, 1993, V 331, N 1, pp. 91-94.
# Shapovalov Yu.B. Modelling of chondrite origin and the interphase distribution of platinum and palladium of liquation iron-sulphide-silicate melts.
The differentiation of iron-silicate melts from which chondrites form, was studied at T=1200oC and P=1 kbar. The experiments were conducted in sealed platinum tubes with inserted graphite crucibles, which provided the reducing conditions of the experiment and the insulation of specimens from platinum tube. The specimens consisted of finely ground picritic glass mixed with chemical reagents (FeO, NiO, elementary sulphur). The bulk composition of two run series differed by the nickel presence. Small sulphide-metal drops were separated in the silicate extremely magnesian matrix in the nickel-free system. All iron was concentrated in these drops, which decomposed into the metallic (significantly ferric) and sulphide-troilite phases. The iron-silicate differentiation was also observed in the nickel-bearing system, but the iron-sulphide drops separating from the silicate melt did not undergo liquation as in the above-mentioned experiment and the heterogeneity of their structure has crystallising origin. The silicate matrix consists of olivine phenocrysts and clinopyroxene-plagioclase groundmass. Its textural relationships of minerals are similar to those in the ordinary LL chondrites, which are characterized by high iron contents of minerals and high nickel content of the metallic phase (Marakushev, Shapovalov, 1995).
The platinum and palladium behaviour was studied at similar experimental conditions. The unusually contrast distribution of these elements between the metal, sulphide, and silicate liquid phases was established. Platinum was almost completely concentrated in the metal phase, which became ferroplatinum with a significant predominance of platinum over iron and containing some palladium. impurity. However, palladium was substantially concentrated in the sulphide phase, which included only a small quantity of platinum. The platinum-palladium partition between the metal-sulphide phases emphasize their affinity to different geochemical groups: siderophile (Pt) and chalcophile (Pd). Therefore, the sulphur content of the considered magmatic systems must determine the platinum-palladium relation in the iron metallic phase. The silicate magnesian melt is almost free from platinum-group metals due to the significant extractive ability of metallic and sulphide melts (Marakushev, Shapovalov, 1996).
References:
Marakushev A.A. The petrogenetic model for the formation of diamond-bearing rocks.
The thermodynamic models were developed for the formation of diamond-bearing rocks in various formations: kimberlite and lamproite pipes (several facies of eclogites and peridotite nodules were established); metamorphic complexes (the relict nature of diamonds was proved); impact ring structures, so-called astroblemes, (their endogenic origin was proved); and meteorites - iron, ureilites, and chondrites (contrary to traditional conceptions, they were proved to have originated early in the planetary evolution under the pressure of the fluid shells of the planets). The diamond formation in all the formation types is associated with magmatic systems with high fluid pressure and is due to fluid-magma interaction. Diamond features, including the carbon isotopic composition, etc., were explained with consideration of variations in the oxidized state of the fluid - CO-CH4-CO2 [1, 2].
The investigation of the formation conditions of the meteorite matter and diamond-bearing terrestrial and lunar rocks included model experiments and microprobe analyses of natural samples (diamond-bearing meteorites - ureilites).
The experimental study of the silicate-iron-sulfide stratification of magmatic systems typical of meteorites comprised the examination of the phase distribution of nickel, platinum, and palladium. Significant distinctions were revealed between the geochemical features of these metals: platinum proved to be markedly siderophile, but palladium - chalcophile. Platinum exhibited the extreme chemical affinity to the metallic melt phase, where it is concentrated almost entirely [4].
The studied natural objects yielded the unique material which made it possible to restore the mechanism of diamond formation in meteorites (diamond's relation to the iron phase in the paragenesis with moissanite, spinel, and graphite). The discovery of pyrope in diamond-bearing ureilites [3] is of great importance. This fact clarifies the formation conditions of the high-pressure assemblage in meteorites and rejects the traditional hypotheses implying the impact origin of the meteorite diamond.
References:
#This study was supported by the RFBR, Grant 95-05-14840.
33
Osadchii Eu.G. and Lunin S.E. Thermodynamics of sulfide systems.
1. Phase diagram and compositions of the coexisting sphalerite-greenockite and kesterite-cherniite solid solutions in the system Cu2SnS3-ZnS-CdS have been studied at T=400oC and P=101.3 MPa. A new mineralogical thermometer has been substantiated [1].
2. Using the e.m.f. method, free energies of dyscrasite (Ag3Sb) and allargentum (AgoSb) formation have been determined in the Ag-Sb system at temperatures below 450oC in the electrochemical cells with AgI as a solid electrolyte. Free energy of miargyrite AgSbS2 ( Go480=119.4 kJ/mol;
Go480=119.4 kJ/mol;  Go502=-118.8 kJ/mol) and pyrargyrite AgSbS3 (
Go502=-118.8 kJ/mol) and pyrargyrite AgSbS3 ( Go493=-285.8 kJ/mol;
Go493=-285.8 kJ/mol;  Go557=-284.0 kJ/mol) formation was determined. Based on these data, phase diagram of the Ag-Sb-S system at 473K was refined.
Go557=-284.0 kJ/mol) formation was determined. Based on these data, phase diagram of the Ag-Sb-S system at 473K was refined.
3. A method of buffering low partial SO2 pressures in the Me-S-O systems and determining thermodynamic properties of phases of constant composition and solid solutions in high temperature galvanic cells with oxygen/ion conductivity of a solid electrolyte has been mastered. A theoretical analysis of the systems metal-sulfide-oxide-silicate was realized which was necessary to study physical-chemical conditions for formation of sulfide ore deposits and meteorites. The possibilities of the experimental study of the said systems by the methods of high temperature electrochemistry were determined. The results obtained are as follows:
- The FeS activities in the sphalerite solid solution have been determined for the compositions 10, 20, 30, and 40 mol% FeS by the method of galvanic cell in the temperature range 850-1020K. Through all the studied temperature range, FeS solid solution in sphalerite has a positive deviation from the ideality [3].
- The ZnS activity in the ZnS-CdS solid solution for the composition of 50 mol% CdS in the temperature range 930-1150K in a special electrochemical cell using a method of "zero potential" has been determined. An insignificant negative deviation of the ideal behaviour of solid solution has been found at XSPZnS=0.5, aSPZnS=0.447 and g=a/x=0.894.
- The same cell was used to refine free energy of pure sphalerite formation, the literature data on ZnS and Cu2S being drawn on. At 1100K  fGoZnS= -156.905+0.175kJ/mol as contrasted to the reference data (Brain,1987) 158.161+0.300kJ/mol.
fGoZnS= -156.905+0.175kJ/mol as contrasted to the reference data (Brain,1987) 158.161+0.300kJ/mol.
4. On an example of diskrasite formation, a technique of high pressure galvanic cell with a common gas space was developed. This type cell offers much promise in the investigation of solid phase reactions at high isostatic pressure and opens up opportunities of direct calibration of geological and cosmic barometers.
We were the first in the world to perform the direct measurements of the volume effect of the diskrasite formation reaction 3Ag+Sb=Ag3Sb at the isostatic Ag pressure 3-8 kbar by the method of galvanic cell.
Pressure coefficient of the cell e.m.f. is (¶E/¶
p)T=1.151.10-3+0.030 mB/atm and in the range studied at T=300-450oC does not depend on temperature. The value obtained ( V=3.384 cm3) agrees satisfactory with the known data for 298.15K and 1 atm. (
V=3.384 cm3) agrees satisfactory with the known data for 298.15K and 1 atm. ( V=3.384 cm3).
V=3.384 cm3).
5. An electrochemical sensor was developed to determine pH2 in high temperature gas mixtures of the C-H-O-S system. pH2 in high temperature fumaroles of the volcano Kudryavy was determined (3.0 mol. at 600oC). Simultaneous determination of pO2, pS2, and pH2 gives an interconsistent geochemical information on gas composition and activities of its main components (pO2, pS2, pH2, pSO2, pH2S, pSO3 etc.). Working time of the sensors in the temperature range 550-700oC is several months and they are well feasible for the geochemical monitoring of the volcanic processes.
6. The experimental study of edge components of argentite-naumannite solid solutions (Ag2S-Ag2Se) has been carried out using the method of electrochemical cells with AgIBr and Ag
7. On the basis of a solid AgI electrolyte in the temperature range 100-450oC and on the basis of a solid electrolyte Ag
References:
Tikhomirova V.I. and Akhmedzhanova G.M. The formation of Au-As intermetallides at low temperatures.
The system Au-As-H2O-HCl was studied at 200-300oC and 50 MPa under different redox conditions. A new phenomenon was discovered - the low-temperature formation of gold-arsenic intermetallic compounds with the general
34
formula AuxAsy, where x = 1-3 and y = 2-6. This phenomenon was first established in reducing hydrothermal solutions, whose hydrogen fugacity was controlled in situ. The stability conditions of the gold-arsenic compounds and aurostibite (AuSb2) in hydrothermal solutions and on air were evaluated. The experiments with variously oriented crystalline gold plates followed by the X-ray spectral and X-ray diffraction analyses of the gold surfaces showed the Au-As intermetallides to form at high hydrogen fugacity (lgfH2 > 1). Under such conditions, metallic arsenic was in equilibrium with arsine (AsH), which interacted with gold through the fluid. At solution acidity no more than 0.1 N HCl, the Au-As compounds occurred as solid solution on the surface of gold. At acidity above 0.1 N and chloride concentration above 0.2 N, the surface gold-arsenic compounds dissolved and evidently yielded complexes, for the gold solubility was higher by a factor of ten (10-6 M) than that in the absence of arsenic at the same oxygen fugacity. As shown by X-ray diffraction analysis, the Au-As compounds, unlike stable aurostibite, decomposed to arsenic oxides of arsenolite type and fine-grained ("mustard") gold when exposed to air or on rising oxidizing potential of the solution. The gold-arsenic compounds were more stable on air if they had formed on the gold surface or in the presence of the third component (Al or Fe, as reducing buffer).
References:
35